The sales & marketing department often aims to create more and more product variants to target even the smallest niche in the market. Yet, it is common wisdom that more variants also mean more inventory.
The sales & marketing department often aims to create more and more product variants to target even the smallest niche in the market. Yet, it is common wisdom that more variants also mean more inventory.
However, the relation is not quite as clear cut. In my last post I wrote about the Relation between Inventory, Customer Takt, and Replenishment Time. The relation is similar for variants, and it all depends on the ratio of the customer takt to the replenishment time.
The Conflict of Interest between Sales and Manufacturing
 There is often a conflict of interest between sales and manufacturing. Sales would like to sell as many products as possible, and ideally would like to have a product for every market niche so no potential customer will be missed.
There is often a conflict of interest between sales and manufacturing. Sales would like to sell as many products as possible, and ideally would like to have a product for every market niche so no potential customer will be missed.
Manufacturing, on the other hand, wants to produce as quickly, easily, and efficiently as possible. Ideally, manufacturing would like to have one and only one product, since that can be made most efficiently.
Obviously, a successful company is interested not only in quantity of sales or cheap manufacturing, but also (and most of all) in profit. Hence, aiming at every market niche but losing money with every sale is not good. Similarly, producing very efficiently but having no market share worth mentioning is not good either. A trade-off is needed.
In this post I will look in more detail at one aspect of this trade-off. How does your inventory change if you change the number of variants produced? Common wisdom is that more variants usually means more inventory for the same quantity sold. That wisdom is true, but let’s back it up with data.
Theoretical Approach
I explained the theory in detail in my last post. The inventory is directly related to the replenishment time over the customer takt, rounded up (and, in reality, you should also have a bit more to consider variation and a safety margin).
\[ Inventory= Roundup[ \frac{Replenishment\; Time}{Customer \; Takt}]\]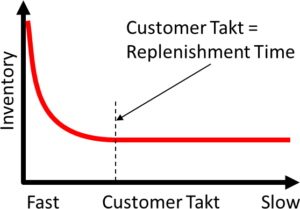 Overall, this will give you a relation for one product as shown in the graph. The inventory is constant if the customer takt is larger than the replenishment time, and increases if the customer takt is faster than the replenishment time.
Overall, this will give you a relation for one product as shown in the graph. The inventory is constant if the customer takt is larger than the replenishment time, and increases if the customer takt is faster than the replenishment time.
Simulation System
 The theory has been tested using the same simulation data as in my last post, using a simple system with one process.
The theory has been tested using the same simulation data as in my last post, using a simple system with one process.
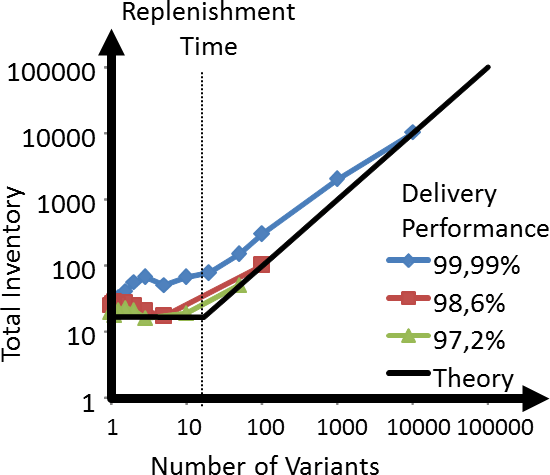
Assume we sell a total of 100,000 items in a year. Now, we could sell all 100,000 items of only a single variant. For example, we produce 100,000 of one variant, or 50,000 of two variants, or 10,000 each of 10 variants, and so on down to 10 items each of 10,000 different variants. We always look at the total inventory necessary for systems with similar delivery performance (99.99%, 98.6%, and 97.2%).
Simulation Results
As mentioned above, the defining element is the ratio between the replenishment time and the takt time. The graph below shows the simulation data and also the theoretical expected value, comparing the total inventory with the total number of variants. Please note that both axes use a logarithmic scale.
The vertical dashed line shows where the customer takt equals the replenishment time. To the left of this line, the inventory stays the same regardless of the number of variants. To the right of this vertical dashed line, however, the inventory increases with the number of variants. Naturally, for a higher delivery performance, you need a higher inventory to account for random fluctuations.
How Do Variants Influence the WIP?
As above, the behavior of the WIP based on the number of variants differs depending on the customer takt and the replenishment time.
- If the replenishment time is faster than the customer takt for an additional variant, then this additional variant WILL increase the inventory.
- If the replenishment time is slower than the customer takt for all additional variants, then these additional variants WILL NOT increase the inventory.
I think this is quite an insight that I have not seen anywhere before. Especially, the distinction of the customer takt being larger or smaller than the replenishment time is new and original research.
This means that you can increase the number of variants with little WIP penalty, as long as the customer takt is faster than the replenishment time. However, if your customer takt is slower than the replenishment time, then additional variants will punish you with larger inventories, whereas reducing variants will also reduce your inventory. In sum, you should eliminate variants if the customer takt is slower than the replenishment time! At the same time, you can introduce additional variants without inventory penalty as long as the variant sells often enough that the customer takt is faster than the replenishment time.
Of course, this looks only at inventory. There are more costs for additional variants. For example, the cost of complexity goes up. You have to stock more and different materials, which increases the work associated with maintaining the material. At the least, you may have to issue two purchase orders when one was enough before. You also have to train your employees in producing these additional variants, and you have to introduce new part numbers. Don’t forget development cost for the new product either!
Also, as for the part numbers, it is estimated that in the automotive industry, an additional single part number will cost around $50,000 over lifetime. For less “rigorous” products like a fridge or washing machine, a new number will still cost around $8,000 over lifetime. So, even if there is no additional inventory, there is still cost associated with every new variant.
Hence, go out, reduce the number of variants, and organize your industry!
Data Source
Meier, Hauke. “Analyse des Zusammenhangs zwischen Variantenvielfalt, Lagerbeständen und Lieferbereitschaft.” Master Thesis, Karlsruhe University of Applied Sciences, 2016.