 The customer takt (or takt time) is one of the fundamentals for determining the speed of a production system. After my post on How to determine Takt Times, this second post on takt times gives a bit of history, and then goes into more details about possible pitfalls and problems when calculating the customer takt. I also added an example for easier understanding.
The customer takt (or takt time) is one of the fundamentals for determining the speed of a production system. After my post on How to determine Takt Times, this second post on takt times gives a bit of history, and then goes into more details about possible pitfalls and problems when calculating the customer takt. I also added an example for easier understanding.
A Brief Bit of History
Takt (German): pulse, stroke, gating, timing, beat, cycle, meter, work cycle

The word “Takt” comes from the German language, since the method was developed in Germany in 1926 in the Junkers aircraft plants. Production of different steps was timed so that the airplanes moved to the next stations at fixed intervals, a method they called Taktverfahren (Takt method). This was not a moving assembly line as with Henry Ford, but a pulse line that moved at fixed intervals (the takt).
This system was also used during World War II in Germany. During that time, about 250 German aircraft engineers and technicians moved to Japan to support the wartime production of their Japanese ally. Through this contact, Mitsubishi learned about the Takt. From Mitsubishi the method spread to Toyota, and hence lean production uses the German word Takt, albeit in a marginally different context.
Please note that when most people talk about customer takt nowadays, they mean the average time between parts. In reality, of course, the time between parts may be quite different. For example in a batch job you may get 100’s of parts at the same time, and then nothing for a long time. The historical takt time above was something set by the people that organized production, and the goal was to keep it constant. Hence, historically the meaning was a bit different from the modern usage of takt in manufacturing.
(Thanks to Joachim Knuf and Michel Baudin for this important piece of history – for more see the highly interesting post 1, post 2, post 3, and post 4 on Michel Baudin’s blog.)
Takt Times for Parallel Processes
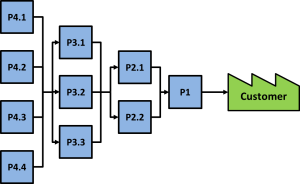
Please keep in mind that the takt will be different if you have parallel processes. In the example shown the first process P1 has the original customer takt. The second parallel processes P2.1 and P2.2, however, have double the customer takt (assuming the processes have an identical speed). Since they work in parallel, each has to do only half of the parts, doubling the customer takt.
\[ \frac{Available \; Work \; Time}{\mathbf{Half} \; Customer \; Demand \; during \; available \; Work \; Time} = \mathbf{Double} \; Customer \; Takt \]Similarly, since each of the three processes P3.1, P3.2, and P3.3 have to produce only one third of the overall quantity, the customer takt triples. Equally for P4.1, P4.2, P4.3, and P4.4 the customer takt quadruples. In general, if you have n parallel processes with identical speeds the customer takt multiplies by n.
If the processes do not have identical speeds, then you would have to calculate each takt separately based on the number of parts that pass through. For example if P2.1 is twice the speed of P2.2, then 2.1 will make 2/3rd of the parts and P2.2. will make the remaining third. The customer takt of P2.1 will then be the inverse of 2/3rd, namely 3/2=1.5 of the original takt, and P2.2. will have 3/1 = 3 times the original takt. But to be on the safe side I recommend to calculate it separately using the number of parts going through each process to avoid errors. Similar is of course also true if the processes have non-identical work times.
Also, be aware if more than one part is needed for the final product. Assume P2.1 and P2.2 have identical speeds, then the customer takt would double. If, however, P2.1 makes the left fender and P2.2. makes the right fender, you would need two fenders per car and the doubled customer takt would halve again to the original value.
It starts to become real tricky if you have three processes making pistons for a five cylinder engine, and one of these processes is 40% slower (Hint: It is 13/25; 13/25; and 13/15 of the original takt). In any case, unless you are really into fractions, go the safe way and calculate the number of parts needed from each process, and then calculate the customer takt for each machine separately.
An Example

Lets make a simple example. We have one manufacturing system that produces two part types, A and B. We have a flexible manufacturing system whose capacity we can adjust by adding or removing workers in the system.
We want to find out how many workers we need to add to the line next week. Hence, your period of interest is next week. We estimate that the customer orders 4.000 products next week, and we have a 5 day workweek with one shift of 7.5 work hours each. This gives us a total available work time of 37.5 hours or 135.000 seconds.
\[ Customer \; Takt \; Next \; Week= \frac{135.000s}{4.000 pcs}=33.75\frac{s}{pcs}\]If for example our workers need 60 seconds to produce one part in average (including machine problems and defects), then we would need at least 1.7 workers to fulfill the customer demand.
For the overall customer takt, we did not care if the worker ordered A or B parts, we were only interested in the total sum. However, if we want to adjust the number of kanban for next week, we would have to analyze part A and B separately. Out of the 4.000 parts total, we predict 3.200 to be of type A and 800 of type B. Hence we calculate the two separate customer takts as shown below.
\[Customer \; Takt \; Next \; Week \; A = \frac{135.000s}{3.2000 pcs}=42.19\frac{s}{pcs}\] \[Customer \; Takt \; Next \; Week \; B = \frac{135.000s}{800 pcs}=168.75\frac{s}{pcs}\]Please note that these takts are larger than the joint takt. These values we can then use for a more detailed calculation of the number of kanban.
Common Mistakes
 When calculating the customer takt please make sure that you do not mix up the numerator and denominator. The customer takt is always a time for one piece, hence the work time is the numerator and the pieces are the denominator. If you mix this up, you calculating the inverse and end up with the throughput – which is also useful but it may no longer match the rest of your formulas.
When calculating the customer takt please make sure that you do not mix up the numerator and denominator. The customer takt is always a time for one piece, hence the work time is the numerator and the pieces are the denominator. If you mix this up, you calculating the inverse and end up with the throughput – which is also useful but it may no longer match the rest of your formulas.
 As for precision, the customer takt is only as precise as your inputs. If you know your future demand precisely down to a single piece, I envy you. Otherwise, make sure you leave some wiggle room in your system in subsequent calculations of capacity (For example, kanbans are more robust and automatically include a wiggle room through supermarket inventories). Also, if your demand prediction is fuzzy, you do not gain more accuracy by making your work time estimate super precise.
As for precision, the customer takt is only as precise as your inputs. If you know your future demand precisely down to a single piece, I envy you. Otherwise, make sure you leave some wiggle room in your system in subsequent calculations of capacity (For example, kanbans are more robust and automatically include a wiggle room through supermarket inventories). Also, if your demand prediction is fuzzy, you do not gain more accuracy by making your work time estimate super precise.
 Finally, while the takt time gives you the average time between parts, the true time between parts will fluctuate widely. A customer may not order a part every 50 seconds, but may order 30.000 on the first of the month and then nothing until next month. The takt time of your process will (hopefully, usually) not fluctuate that much, but even there most parts will be much faster than the average, but every now and then a long delay or breakdown will be much, much slower than the average.
Finally, while the takt time gives you the average time between parts, the true time between parts will fluctuate widely. A customer may not order a part every 50 seconds, but may order 30.000 on the first of the month and then nothing until next month. The takt time of your process will (hopefully, usually) not fluctuate that much, but even there most parts will be much faster than the average, but every now and then a long delay or breakdown will be much, much slower than the average.
I hope these two posts on takt times were helpful for you. In the next posts I will introduce more important times. In the meantime go out and organize your industry!

Very informative article about takt time calculation. In section, “common mistakes”, i believe the word ‘numerator’ has been mistyped as ‘nominator’. Please check and make necessary correction.
Hi Ashok, you are right! This was a gap in my English skills (non native speaker). Thanks for the update!
Very informative and easy to understand . The examples are really very much explanatory.
Thanks u sir.
Hi Chris,
Regarding the example above about type A and type B. When you did the calculation, do you mean both parts are produced on same line or 2 parallel lines? Can you elaborate please?
Hi Mo, the would be on the same line. But you can also see it from the customer side. The customer wants in average one part A every 42.19s, one part B every 168.75s, or combined one part every 33.75s.